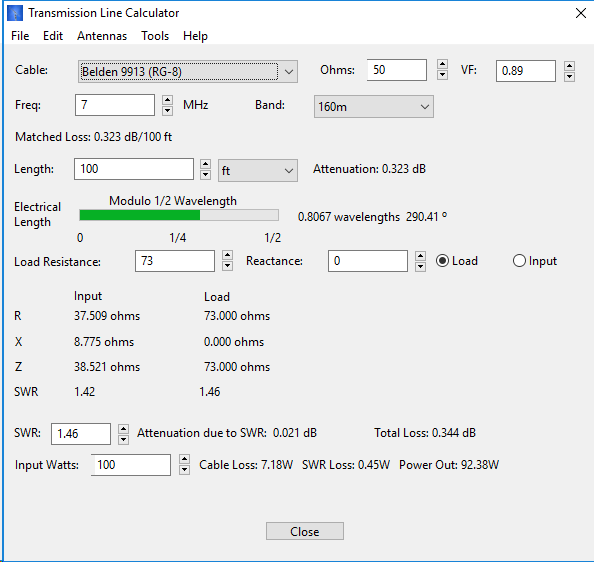
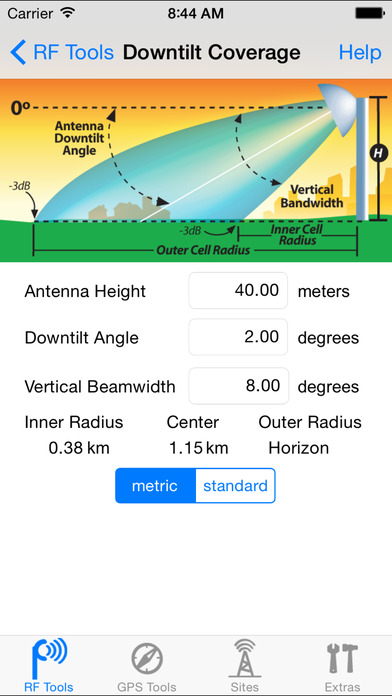
TLV (Tag, Length, Value) byte strings into their constituent tags and sub-tags. (there's a version for encoding as well)Ĭonverts ASCII to hex and vice versa, as well as to other number-based encryption methods.Įverything you need to know about Chaffing and winnowing.ĮMV tag search. Made by Discord users in Team Look In The Bushes.ĭecodes ascii85 messages.
Originally forming part of an ARG, the site now houses two series of puzzles - the originals, and a cancelled Advent version. Will put your basic puzzle solving skills to the test. Levels can be gained through active participation on the official forum.Ī short, interactive, slightly creepy story made by Discord user mai(#9088). They begin as really easy, but then ascend to a more difficult encounter.Ī series of puzzles designed to introduce people to ARG solving. Created by the GameDetectives admins.Ī puzzle site that has many categories of learning. Made by Discord user crashdemons.Īn annual Game Detectives tradition consisting of a series of puzzles unlocking each day over advent, with varying difficulty. They get more difficult the higher you go.
3.6 Kansas City Standard Cassette Audio DataĪ series of challenges meant to teach to basic elements, techniques, and dynamics of ARGs, made in-house by the Game Detectives.Īe27ff is a series of puzzles that use the typical techniques of most ARGs. 2.19 Linux Command Line Encode / Decode. Part of the "Computed Tomography and the ASTRA Toolbox" course. Analytical projection (the Radon transform) (video). Natterer, Frank Wübbeling, Frank (2001), Mathematical Methods in Image Reconstruction, Society for Industrial and Applied Mathematics, ISBN 0-89871-472-9. Natterer, Frank (June 2001), The Mathematics of Computerized Tomography, Classics in Applied Mathematics, vol. 32, Society for Industrial and Applied Mathematics, ISBN 0-89871-493-1. (2001), "Radon transform", Encyclopedia of Mathematics, EMS Press (2009), Fundamentals of Computerized Tomography: Image Reconstruction from Projections (2nd ed.), Springer, ISBN 978-1-85233-617-2 Helgason, Sigurdur (2008), Geometric analysis on symmetric spaces, Mathematical Surveys and Monographs, vol. 39 (2nd ed.), Providence, R.I.: American Mathematical Society, doi: 10.1090/surv/039, ISBN 978-0-8218-4530-1, MR 2463854. (1983), The Radon Transform and Some of Its Applications, New York: John Wiley & Sons Integral Transforms and Their Applications. Lokenath Debnath Dambaru Bhatta (19 April 2016). "A short introduction to the Radon and Hough transforms and how they relate to each other" (PDF). Tomographic Reconstruction of SPECT Data. "Applied Fourier Analysis and Elements of Modern Signal Processing – Lecture 10" (PDF). "Applied Fourier Analysis and Elements of Modern Signal Processing – Lecture 9" (PDF). Helgason, Sigurdur (1984), Groups and Geometric Analysis: Integral Geometry, Invariant Differential Operators, and Spherical Functions, Academic Press, ISBN 0-12-338301-3. If a function f : CS1 maint: ref duplicates default ( link). The Radon transform is widely applicable to tomography, the creation of an image from the projection data associated with cross-sectional scans of an object. The complex analogue of the Radon transform is known as the Penrose transform. 
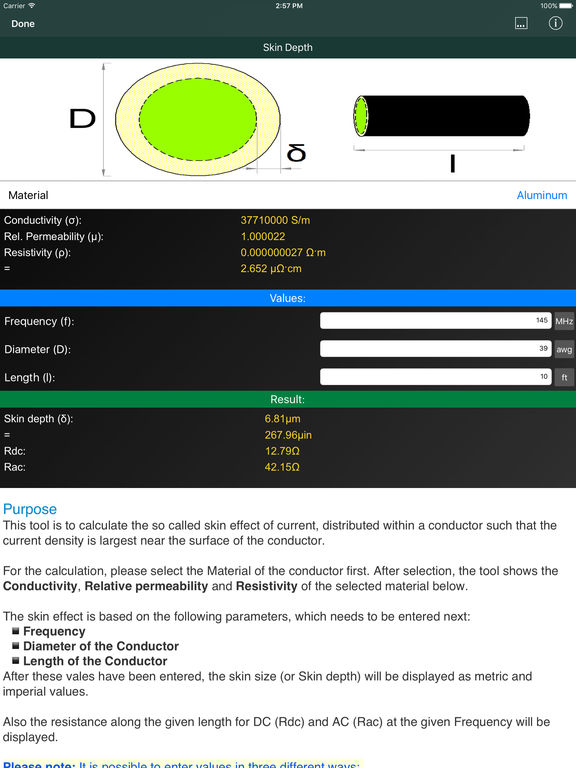
It was later generalized to higher-dimensional Euclidean spaces and more broadly in the context of integral geometry. Radon further included formulas for the transform in three dimensions, in which the integral is taken over planes (integrating over lines is known as the X-ray transform). The transform was introduced in 1917 by Johann Radon, who also provided a formula for the inverse transform. In mathematics, the Radon transform is the integral transform which takes a function f defined on the plane to a function Rf defined on the (two-dimensional) space of lines in the plane, whose value at a particular line is equal to the line integral of the function over that line. Original function is equal to one on the white region and zero on the dark region.
0 kommentar(er)
